Solution to the physics problem I found

You may have noticed that as object A moves to the left there are several variables that change with respect to time. The vertical height y increases, the horizontal length x decreases, and the angle alpha increases. This is really no different from an ordinary related rates problem, so we'll treat it like one.

Start out by using the Pythagorean theorem to write the vertical length y as a function of the horizontal length x.

recall that the derivative of displacement with respect to time is equal to velocity. So in order to create a velocity equation, we will differentiate both sides of the equation above with respect to time.

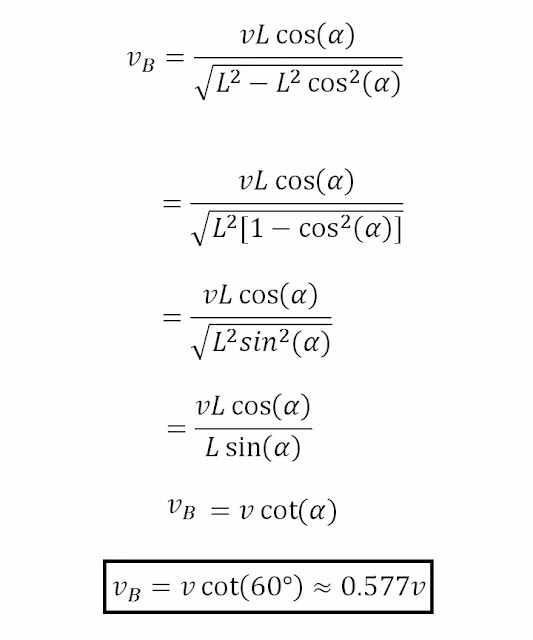
We're almost ready to solve the problem. All we have to do now is a little bit of substitution and manipulation of the problem.

important note* dx/dt is the velocity of A. It's important to know that the velocity of A is going to be negative because as A is moving to the left, the length of side x gets smaller. This means that the rate of change of x with respect to time (dx/dt) is negative. It is thus substituted with negative v.

at this point it's just a matter of rearranging parts of the equation and making it look nice. It should simplify to something fairly simple where we can plug in the angle alpha.

Isn't it mind blowing? We started out with a nasty looking velocity equation and simplified to something simple.

A cool physics problem I found

I found this problem in my physics book. It really stumped me for a while, but I finally figured it out after some time. It looked intimidating, but it's not as bad as I thought. I tried to redraw the diagram as closely as I could and I almost copied the problem down word for word.

Two objects, A and B, are connected by a rigid rod that has a length L. The objects slide along perpendicular guide rails, as shown in the figure. If A slides to the left with a constant speed v, find the velocity of B when the angle, alpha equals 60 degrees.

Solution to the cool integration by parts problem

This problem requires a bit more ingenuity.

Start out using integration by parts as you normally would.

You may notice that the new integral acquired still poses the same issue we started out (but with cosine instead of sine). Let us do integration by parts once more and see what happens

Now I need to substitute this back in. I need to also make sure to distribute the negative.

Now we have the exact same integral we started out with on both side. all we really need to do is add the integral of e^(x)sin(x) to both sides of the equation. Then we'll have 2 times the integral of e^(x)sin(x) on one side. Simply divide by two and the whole thing will be solved.

Is your mind blown or what?

A cool integration by parts problem

Here's a cool integral that requires integration by parts. It's a little tricky, but the solution is mind blowing.

Deriving the volume of a sphere solution

note* I'm assuming you are already familiar with calculus. If you're not, it's probably not going to make any bit of sense.

I will be doing this using integration techniques. Remember learning about solids of revolution from calc. I? That's what I'll be using. I'm not really sure if it's the best way, but it works.

Consider the graph of a basic semi-circle. If you were to revolve it around the x-axis, the solid generated would be a sphere whose cross sections are circles.

Imagine slicing through a sphere. You'll notice that the cross sections are circles of varying sizes. What I'll essentially be doing is creating a summation of disks that each have a very small thickness of dx.

To start off, I'm going to create a function of the cross sectional area and integrate it from one end of the semi circle (-r) to the other (r). I'll need the formula for the area of a circle. The radius of each cross section will vary as a function of x, and so I need to make the appropriate substitution for the radius. Through integration, the disks of varying cross sectional area will be summed together to create a sphere.

There's nothing all that tricky about solving this integral. If you work it all out you should end up with the correct formula for the volume of a sphere.

Deriving the volume of a sphere

For this next one I want to derive the volume of a sphere using calculus. I don't have much more to add, but I feel like I should at least post a picture of something...